Stefan's Tesla-Pages
Technological background, part#4
(or 'how to produce your own indoor lightning')
![[home]](buthome.jpg)

Some definitions and some descriptions of "how to measure":
-
coupling (k-factor, coupling coefficient k)
quenching
quality factor (Q)
k-factor
(coupling)
-
Definition:
-
k is defined by the formula:
k = M / sqrt(L1 * L2)
where L1 and L2 are the inductances of the primary and secondary coils and
M the mutual inductance between both coils.
-
-
The coupling factor k determines how quickly the energy is transferred between
the primary coil and the secondary coil (and back). It is almost entirely
determined by the geometry and physical positioning of the two coils relative
to each other. If the coils are spaced far apart, the coupling is "loose"
and the k-factor is low. Energy transfer between the coils is slow and takes
many RF cycles. A tighter coupling means higher k and faster energy transfer.
Typical coupling coefficient for standard Tesla coils are in the range between
0.05 and 0.2. The looser the coupling, the shorter the sparks. But if the
coupling is to tight, there will be racing sparks along the secondary and
breakdown between the primary and the secondary. High voltage RF is VERY
difficult to insulate, especially along insulating surfaces. An RF voltage
that may jump only 1" in air, can easily flash over 3-4" along the surface
of a dielectric. You should build you TC so that you can raise the secondary
relative to the primary to bring coupling within the proper range. Start
with the secondary elevated (low coupling), and tune for best spark before
going to full power. Then you can slowly increase coupling to get best full-power
operation without racing sparks.
-
-
Measurement 1:
-
A very easy way to measure the coupling was presented by Dan Kline in
his mail on the TCML on 03.09.1998. For his method you only nead a 10kOhm
resistor, a 1uF capacitor, a "household power resistor" like hair dryer
or space heater and a multimeter for AC current (approx. 10A) and AC voltages
(~1V). You'll get the mutual inductance and calculate k with the knowledge
of the inductances L1 (primary coil) and L2 (secondary coil). But now
with his own words:
-
-
-----------------
Subject: coupling coefficient - Best Method
Date: 03.09.98 03:13:39 CETDST
From: tesla-at-pupman.com (Tesla List)
To: tesla-at-pupman.com
-
Original Poster: Dan Kline <SNIPed>
-
All,
I have tried all the suggestion I have received (Thanks Malcolm, Fr. Tom,
John C., Mark Rzeszotarski). The best method I have found that does not require
expensive equipment or great theoretical challenges consists of the following.
-
Apply a heavy 60 Hz AC current to the primary coil. This is best done by
placing a space heater, hair dryer, etc. in series with the primary to limit
the current to about 10 amps. Measure this current with a multimeter. Note
that the space heater gives a fairly stable resistance. Light bulbs have
a non-linear resistance through the AC cycle and distort the measurement
(they must cool down substantially at the nodes of the AC cycle). Of course,
use great caution with the live AC on the primary so as not to kill yourself.
Only the isolated primary need be connected to the AC. The capacitors,
transformers, and other wiring should be disconnected from the primary for
this test. Be cautious of the AC finding its way on to the secondary!
-
Place a 10k ohm resistor and a 1uF capacitor across the secondary and measure
the AC voltage. It will be on the order of say 100 mV AC. The resistor and
capacitor will eliminate stray noise picked up by the secondary and
swamp any resonance which is significant at these low levels.
(Remark: resistor and capacitor in series or in
parallel?)
-
The mutual inductance is found by:
-
M = V / (w * I)
Where:
-
M = Mutual inductance in Henries.
w = the line frequency in radians per second (377 for 60Hz
or 314 for 50 Hz).
I = The measured current in the primary in amps AC.
V = The measured secondary voltage in volts AC.
-
As an example:
If the current in the primary is 10 amps and the frequency is 60Hz and you
measure 0.100 volts AC, you would get:
-
0.100 / (377 * 10 ) = 26.52 uH for the mutual inductance.
k can then be found by using the formula:
-
k = M / sqrt(L1 * L2)
Where L1 and L2 are the inductances of the primary and secondary coils.
-
This method is rock solid in theory and easy to do. The accuracy is excellent.
There is little that can go wrong compared to other methods and you don't
need anything special other than a multimeter to do the test. The accuracy
is dependant on the accuracy of your multimeter. My tests could easily get
within 1%.
-
-
Measurement 2:
-
This method requires a storage scope. You can determine the coupling simply
from a scope trace showing the ringdown of the secondary circuit - if you
have a storage scope available. The waveform (small signal, no heavy sparking
from secondary!) is an amplitude modulated sine wave with some "beating"
at the beginning (where energy is tranferred back and forth between the
primary circuit and the secondary circuit) and an exponential decay
at the end. Take a closer look at the section between two notches of the
beating at the beginning of the waveform. Count the number (n) of full sine
cycles inbetween those two notches. Coupling factor k simply can be derived
by k=1/n.
-
-
For instance, if in the first ringdown you can count 5 "sines" (one local
max (high) plus one local minmum (low) each), then k=1/5=0.2:
-
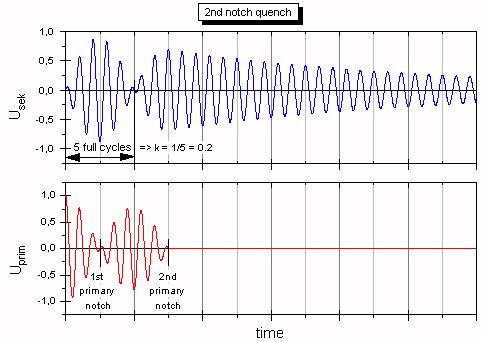
-
The approach of estimating "k" by taking the reciprocal of the number of
peaks during the first primary ringdown is accurate to within 5-10% for the
typical range of "k's" used in 2-coil systems.
-
-
Measurement 3:
-
Measure fI and fII of the secondary, with fI
= primary shorted and fII = primary open circuit. As defined above,
k is ...
 (still have
to find again where I've read about this method...)
(still have
to find again where I've read about this method...)
-
-
-
'Magic' k-factors:
-
Sometimes you might hear about 'magic' k-factors. Forget them! The
theory is based on very simplyfied circuits and therefore those 'magic' k-factors
simply are wrong...
-
Quenching
-
-
Definition:
-
"Quenching" is the ability of the spark gap to stop conducting. This
can only happen when the energy (both current and voltage) in the primary
circuit is low for a significant amount of time. So this will only happen
at the primary notches described above. If there are enough ions in the air
between the gap, it will re-ignite if the voltage rises again and will quench
at a later notch.
-
-
What is "good" about an early quenching?
-
The goal is to achieve a "good" quenching, that is an early quenching (preferable
the first or second notch) so that no more power is dissipated in the spark
gap as heat. The reasons for that are:
1) The heat errodes the electrodes.
2) The heat reduces the firing voltage of a static gap so that less energy
could be transferred to the secondary.
3) An early quench reduces the average current in the primary circuit. Therefore
the gap and capacitor are stressed less. Compared to a 4th notch quench
(exponential decay neglected), a 2nd notch quench reduces the average current
down to only approx. 3/7 (43%), a 1st notch quench even down to 1/7 (14%)!
-
-
Measurement:
-
You can observe the quenching on a storage scope. Simply use an antenna far
enough from the secondary (so that it doesn't get hit).
-
-
How to achieve a good quenching:
-
1. Run a cool ( not necessarily fancy ;-) gap
The gap should have a big thermal mass and good cooling by a sufficient forced
airflow which also helps to blow away the ions.
2. Use a high primary voltage
A high voltage (10-20kVeff) allows a smaller primary capacitance and (this
the important one) a higher primary inductance. This high inductance increases
the surge impedance and reduces the current in the primary circuit (=>
less heat).
3. Be sure your tuning is exact
If you run the TC for the first time, you should not apply power for long
times, just enough to do the fine tuning (frequency will change with the
length of the sparks!). If your tuning is bad, not all the energy will be
transferred to the secondary and the notch will not be a real notch since
the envelope of the current waveform does NOT fall to zero! It would be very
difficult to get a quench in this case.
4. Allow spark breakout
Of course, a toroid never can be to big. But a big toroid can prevent spark
breakout. Therefore, a breakout point should be used (at least at the beginning
unless the system is set up perfectly, then it can be removed later) at the
toroid. This is to remove the power from the system as quick as possible.
If you use a variac to slowly bring up the voltage without the use of a breakout
point, you might not get breakout though the spark gap is firing until you
cranked it up to a certain level. In this situation, all the power is dissipated
in the gap and primary capacitor, a very destructive situation!
Q (quality
factor)
-
-
Definition:
-
Q = XL / RAC = (2*Pi*Fres*L) / RAC
-
with
Fres = resonance frequency of the coil
RAC = resistance of the coil at resonance frequency
(>RDC because of skin effect and proximity effect)
-
-
another definition:
-
-
Q = (Fres/BW )
with
BW: Bandwidth of the coil
-
-

-
Goals:
-
Make the primary Q as high as possible. This means high inductance
(and low RAC). The impedance of the primary coil usually is at
least one order of magnitude higher (approx. 20-40Ohms) than the resistance
of the primary coil and of the spark gap (up to 2Ohms). Therefore the primary
current will be lower for higher Q coils and the losses in the sparkgap will
decrease. Spark gap losses reach up to a sifgnificant amount of the
input energy, so this is really the place to look at! If the secondary is
sparking well, the primary Q of the live TC is in the order of 10.
The secondary Q will decrease dramatically if the sparks break out
and it will go flatline if you have an power arc to ground. This means
that for spark gap (!) driven coils it is useless trying to achieve a
very high secondary Q (please read this sentence again!). Instead of
this, its better to wind more turns of thinner wire for higher inductance
because this will result in a higher primary inductance with the advantages
mentioned in the paragraph above. So there is a compromise between
inductance and resistance. High Q is required when the voltage in the secondary
swings up before breakout. When the coil is sparking and when an arc is formed
to ground (resulting in a closed circuit), the AC resistance should also
be small. I would say go for about 1500-2000 turns in the secondary of spark
gap driven coils.
OK, now lets have a look at CW-TCs [continious wave TCs like vacuum tube
driven Tesla coils (TTTCs) or solid state driven Tesla coils (SSTCs)]. Here
the secondary voltage is not defined by the energy dumped into the secondary
at one shot but on the resonant rise due to continious energy transfer. If
you shoot for a very small wire to achieve a high inductance of the secondary,
the DC-resistance will increase dramatically. Also the AC-resistance (skin
effect, proximity effect) will do. Q should be high here, go for thicker
wire! As a rule of thumb, you should shoot for about 500-800 turns in the
secondary coil of a CW Tesla coil. Else, you'll get a serious heating problem
(once in a while, you can read about molten coil formers).
If you place a topload onto your secondary, the Q will drop a bit.
-
-
Measurement 1:
-
To measure the Q of a TC secondary, you'll need an o'scope & an f-gen.
You'll get Q and fres from this measurement. This method was purposed by
RWB on the GTL in 1999.
-
Setup:
-
Place the secondary coil (without primary coil!) in the middle of an open
space in your lab (the most difficult part ;-) well away from anything which
could influence the measurement. It should be elevated so that eddy currents
in your conductive floor (concrete) and ceiling are minimized. Attach a wire
(will act as an antenna) approx. 1m from the top end of your secondary (i.e.
hang it froom the ceiling). Connect this antenna with a coax cable to your
o'scope without using the o'scopes ground. The "hot line" from the
f-gen has to be connected to the bottom end of the secondary. You have to
use the low impedance output from the f-gen here! The f-gen ground will be
left unused, too. Connect the f-gen to the second channel of the o'scope.
-
Measurement:
Set the f-gen to the calculated resonance frequency of the secondary.
You'll get a small signal from your antenna at channel one on the o'scope.
Adjust frequency until you get maximum amplitude, then you've found
the real resonance frequency (will differ some percent from the calculated
value). It is important to stay well away from the coil so that you don't
disturb the measurement. Set the Volts/div so that you'll see this maximum
amplitude well. Write down the exact resonance frequency and amplitude. Also
write down the amplitude of the signal you feed from the f-gen into the secondary
coil. Now adjust the frequency so that you'll get exactly 70.7% of the peak
amplitude. Do this for the higher and the lower frequency (both sides of
the resonance frequency). Write down those two frequencies as well as
the amplitude of the signal you feed from the f-gen into the secondary coil
in each case. Repeat all those measuements at least three times. It is difficult
to adjust the frequency and read all those values with the required precision,
please take time and a good set of instruments. If your f-gen does not provide
sufficient readout, a separate frequency counter is required.
Important: If the signal fed into the coil varies in amplitude (due to
the changing load the secondary presents near resonance), the output impedance
of your f-gen is to high and you can't do the measurement this way! You'll
get a to low Q since the 70.7%-values present a smaller load and the high
and low frequency will move to higher resp. lower
values.
Calculation:
For the calculation, you first have to check if the measured values of the
different measurements are confident. The calculate the mean values. The
final calculation goes like this (example, values from RWB, bare secondary
without topload):
Fres = 227.459kHz
Fhigh (0.707*max.Amplitude) = 228.072kHz
-
Flow (0.707*max.Amplitude) = 226.915kHz
Bandwidth BW = Fhigh-Flow = 1.157Khz
Q= (Fres/BW) = 227.459kHz / 1.157Khz ~197
-
-
The described procedure is not easy and requires a very good equipment, therefore
I want to present another one.
-
-
Measurement 2:
-
For the second method to measure Q of a TC secondary, you'll need an o'scope
, an f-gen and a 10 turn non inductive variable resistor (approx. 2kOhms).
You need to know L before and you'll get Q and fres. This method was purposed
by RWB on the GTL in 1999, too.
-
Setup:
-
Use the same setup as in the method described before. Connect the variable
resistor between the f-gen and the secondary.
Measurement:
Adjust to resonance and set the output of the f-gen to an exact amplitude.
Now adjust the resistor so that you'll have exactly 50% of that amplitude
across the resistor. Re-adjust the frequency and resistance until you have
exactly 50% of the amplitude at really the resonance frequency. Disconnect
the variable resistor and measure to what value you've set it. This value
is the same a the AC-resistance of the secondary at resonance frequency,
because we've buildt a voltage devider (secondary and resistor) and
adjusted for equal voltages. The AC resitance of the secondary will
be multiple its DC resistance, so be sure you use a resistor of sufficient
resistance.
Calculation (example again with values from RWB):
L = 35.5mH (measured before, e.g. with LCR meter)
-
Fres = 227.459kHz
RAC at Fres (read out from variable resistor) = 264.24 ohm
-
(for comparison: RDC = 17.3 Ohm, so
RAC~15*RDC!)
With the formula
Q = XL / RAC = (2*Pi*Fres*L) / RAC ,
-
this results in a Q of:
Q = (2*Pi*227.459*10^3*35.5*10^-3) / 264.24 = 50735.43 / 264.24 ~ 192,
that's nearly the same as before.
This second method is much simpler and faster but not as accurate as the
first one (difficult to adjust the variable
resistor).
-
-
back to the top
-

-
![[home]](buthome.jpg)


